Colour Match Puzzles

Colour Match Puzzles

All images © Abaroth. Permission is given to reproduce for non-profit purposes only.

Home Historic Sites Models Heraldry Puzzles Garden Links

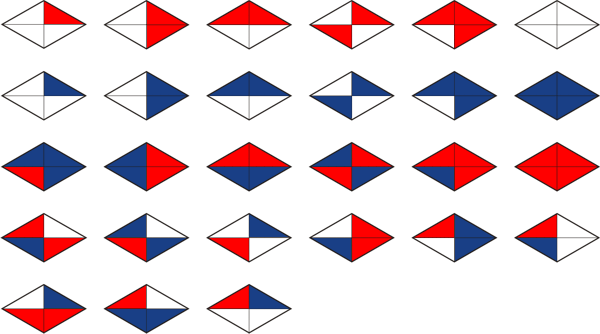
Several different puzzles can be made using copies of the same tile with different colourations.

You could simply paint the patterns on 1" tiles, or use individual triangles for the first set and 1/2" squares for the second.

Make 24 square tiles and colour their edges in every permutation of 3 colours, as shown below. This set of pieces is often called the MacMahon Squares. Kadon Enterprises makes a set of these tiles.		Make 24 square tiles and colour their corners in every permutation of 3 colours, as shown below. Kadon Enterprises makes a set of these tiles.

Puzzle 1: Fit the 24 pieces into the grid below so that all edge colours match. Hover over the grid below for a solution:	Puzzle 2: Fit the 24 pieces into the grid below so that all edge colours match, but with the perimeter of the rectangle in a single colour. Hover over the grid below for a solution:	Puzzle 1: Fit the 24 pieces into the grid below so that all edge colours match. This puzzle is harder than the MacMahon puzzles due to having two colours on each edge. Hover over the grid below for a solution:

	A set using four different colours contains 70 pieces as shown below. Kadon Enterprises makes a set of these tiles.

Equivalent pieces can be made from polyominoes, with each colour represented by a different interlocking pattern, as shown below. The pieces are not allowed to be turned over. 36 unit squares can be made to represent four different colours.	Solution using a set of polyomino pieces with 3 different edge designs.	Similar sets can be made from polytans (pieces constructed from right isosceles triangles). Again, the pieces may not be turned over.

More MacMahon Musings

I thought that I could easily make polyiamond and polyhex versions of the same pieces, but soon discovered that I was wrong. Skewing the square to make a rhombus means that obtuse and acute versions of the same cycle of colours are needed in some cases. I found that if I allowed the pieces to be turned over, there were 27 tiles which can form a hexagon and other symmetric patterns.

More information about this new puzzle may be found at Abaroth's Rhombi

Kate Jones from Kadon Enterprises contacted me to say their Grand Snowflake puzzle was equivalent to the "MacMahon" Squares in four colours. The puzzle is shown to the right - click it to link to Kadon's page. After some thought, I discovered it was not the same, since the pieces with heart-shaped connectors connect to the opposite "colour". The 4 +hearts piece can sit next to the 4 -hearts piece. This got me thinking that there must be a third variation on the puzzle. The piece connections for each are shown below

"MacMahon" Squares: A --> A, B --> B, C --> C, D --> D

Grand Snowflake: A --> A, B --> B, C --> D, D --> C

Abaroth's Squares: A --> B, B --> A, C --> D, D --> C

The puzzle can be solved with no restriction as to which pieces make up the edges. I will post any further information regarding this latest idea as I find it.

Home Historic Sites Models Heraldry Puzzles Garden Links

Contact me with suggestions, comments or questions.